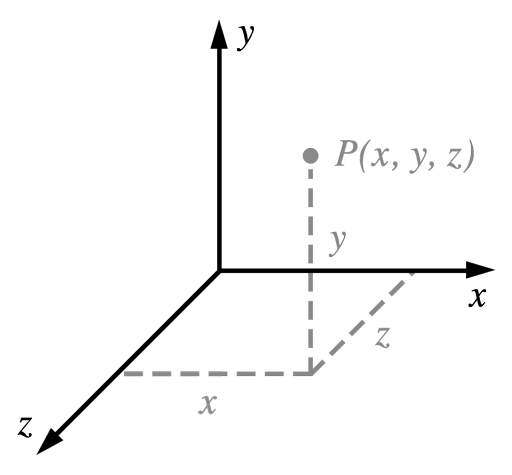3d Vector Right Hand Rule

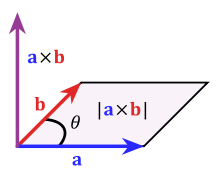
The cross product of two vectors a and b is defined only in three dimensional space and is denoted by a b.

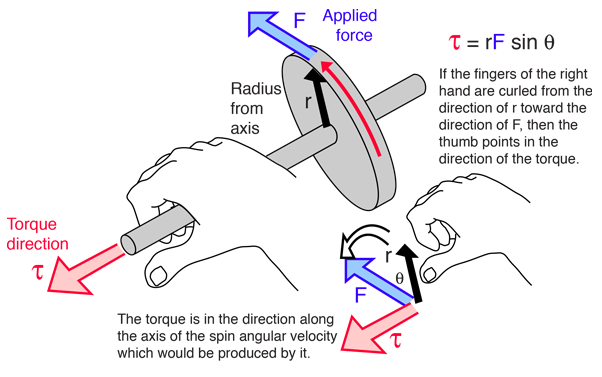
3d vector right hand rule. It is conventional to choose it in the right hand rule direction along the axis of rotation. In physics the notation a b is sometimes used though this is avoided in mathematics to avoid confusion with the exterior product. Area of parallelogram via cross product. Part of the torque calculation is the determination of direction.

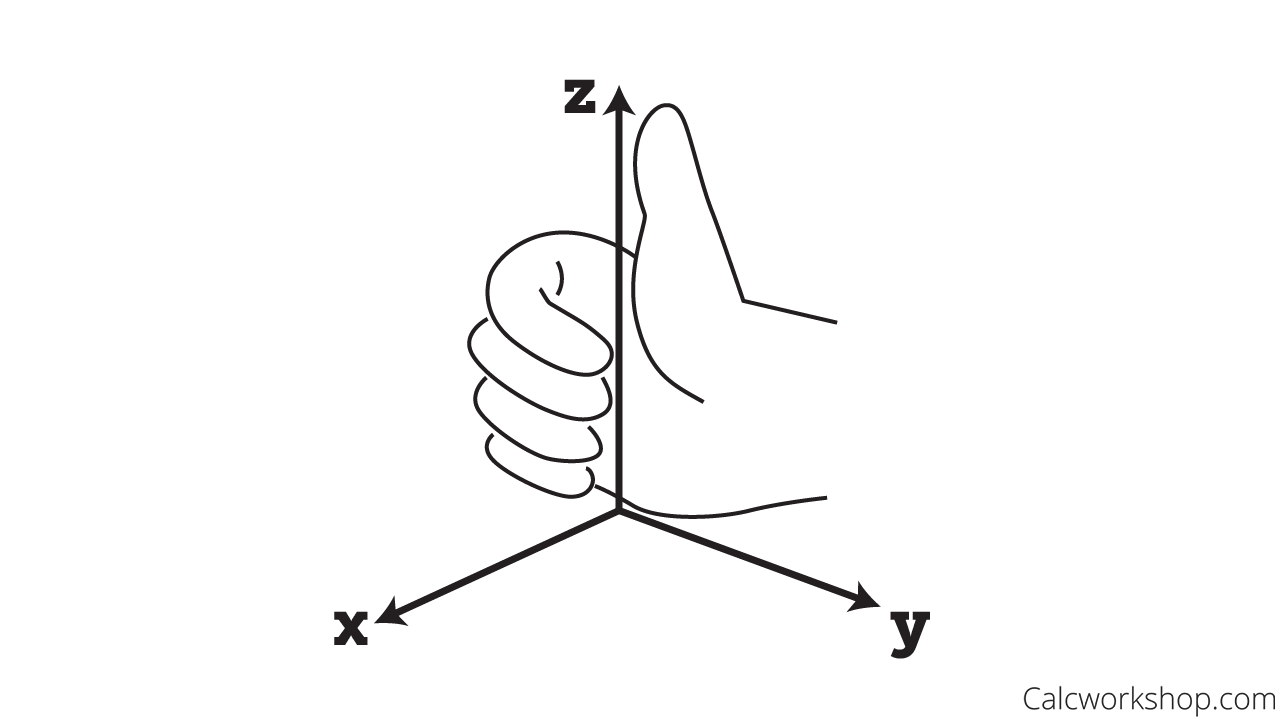
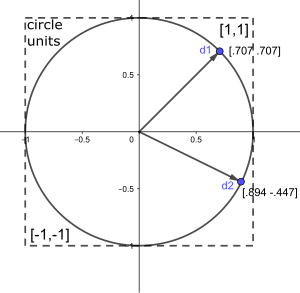
Base vectors for a rectangular coordinate system. Its orientation is determined by the right hand rule. Using the right hand rule to find the direction of the cross product of two vectors in the plane of the page. The projections of vector a along the x y and z directions are a x a y and a z respectively.

Finding the direction of the cross product by the right hand rule. The direction is perpendicular to both the radius from the axis and to the force. D left the right hand rule used to find the direction of e a b and right the right hand rule used to find the direction of e b a. Point your thumb in the direction of the magnetic field this time and curl your fingers just as before.

B the vector difference a b a b d. In mathematics and physics the right hand rule is a common mnemonic for understanding orientation of axes in three dimensional space. Right hand rule the right hand rule is a visualization technique used to determine the correct direction of a vector resulting from vector cross product multiplication. A coordinate system represented by base vectors which follow the right hand rule.

A the vector sum c a b b a. A vector of unit length. Torqueis inherently a vector quantity. Its length is equal to the area of the parallelogram determined by both vectors as seen in the images below.

A set of three mutually orthogonal unit vectors right handed system. Rectangular component of a vector. It is based on the following sign convention for an xyz coordinate system as shown below. One can see this by holding one s hands outward and together palms up with the fingers curled and the thumb out stretched.

The right hand rule applied to a coiled wire. This time the circular direction of your fingers tells you the direction of the current that creates the magnetic field.